Calculus: Infinite Limits vs Limits at Infinity with Explanations, Practice Questions, and Answers [AP Calculus, Calculus 101, Math]

By Hannah Gao on 14 Jun 2022 • ( 2 Comments )

Recall that limits can be written in the form

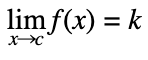
where c and k are constants, and f(x) is a function.

You can, however, have limits that are evaluated at infinity or have an evaluated value of infinity. While infinity is a strange concept, we can use it to determine the behavior of functions. This leads us to the discussion of infinite limits and limits at infinity.

Infinite limits

Infinite limits are limits that evaluate to infinity. They look something like:

Basically, what this says is that as x approaches some x-value, c, the function f(x) blows up in the positive direction (given by the positive infinity) or blows up in the negative direction (given by the negative infinity). In other words, the function is getting super large in the positive or negative direction without bound.

While infinity is not technically a “value” that f(x) is approaching, we can imagine it as such. In general, if a limit evaluates to infinity, the limit would not exist; however, by writing it out as

instead of merely stating “the limit does not exist,” we provide a lot more information about the behavior of the function at a certain value.

Usually, you will see one-sided limits when dealing with infinite limits. This is because infinite limits generally imply some kind of vertical asymptote, which are described separately from the left and from the right. Asymptotes are a type of discontinuity and are very important for analyzing the behavior of functions.

Here’s how you can tell what type of behavior you have at some x-value, c, of a function using one-sided infinite limits. Note that each of the following shows asymptotic behavior.

If you’re wondering just how a limit can evaluate to infinity (or in other words, how we can create an asymptote) just take a look at the function f(x) = 1/x. Imagine what would happen to the graph of the function as x got really close to 0. That’s right – the denominator would get super small, thus making the fraction 1/x super large. As you take smaller and smaller values of x close to 0, the values of f(x) will grow quickly without bound, generating an asymptote. Of course, whether the values go to positive or negative infinity depends on which side we are approaching 0 from, which shows why one-sided limits are extremely important when dealing with infinite limits.

As you can see, when we have a denominator get super close to 0, we can (but not necessarily) have an infinite limit, for our fraction gets super large without bound. Note that asymptotes apply mostly to rational functions (whose numerator and denominator are polynomials with degree greater than 0), and do not show up in standard polynomials. This is because polynomials don’t have anything that causes them to “blow up” to infinity or negative infinity, but many rational functions have denominators that do.

There is a general procedure for finding asymptotes for rational functions:

factor the numerator
factor the denominator
cancel any common factors in the numerator and the denominator
set the denominator to 0

The reason we factor the numerator is because we want to make sure nothing cancels. For instance, if we had an (x-3) term in the numerator of a rational function and a (x-3) in the denominator as well, then these would cancel, creating a “hole” at x =3, but there would be no asymptote! Next, we must factor the denominator and set each factor equal to 0 to find the x-values that will make the denominator 0. As x approaches any of these values, the denominator will get infinitely small and the rational function infinitely large.

Limits at infinity

Don’t be fooled – limits at infinity are not the same as infinite limits. While they both are some kind of limit and have something to do with infinity, they have different meanings and applications.

While infinite limits evaluate to infinity, limits at infinity are evaluated at infinity. Now this might sound strange, because infinity is not technically a “value” at which you can evaluate a limit. However, we can treat limits at infinity as an analysis of a function’s end behavior. In other words, it answers the question “As the values of x get larger (either in the positive or negative directions), what happens to the y-values of the function?”

Note that we are not actually evaluating our function at infinity but rather thinking about our function as x approaches larger positive or negative values.

When we say limit at infinity, we mean one of two things:

The limit on the left is looking at f(x) as x gets larger and larger in the positive direction, thus analyzing the right-ended behavior of the function. On the other hand, the limit on the right analyzes the left-handed behavior of the function, as it is looking at f(x) as x gets larger in the negative direction.

So, what is the purpose of evaluating a limit at infinity? What does it tell us exactly? As we mentioned earlier, limits at infinity help us look at the end behavior of functions. The end behavior of a function f(x) is the behavior of its graph as the values of x approach infinity and negative infinity. You may have heard the term “end behavior” used in previous math courses when looking at polynomials, and you were probably told to look at the leading coefficient and degree to determine end behavior. What you did then was essentially using limits. Let’s take a look at an example why.

Determine the end behavior of P(x) = 3x⁴ + 3x³ + 2.

Before, you would probably look at this and say “The polynomial has an even degree, namely 4, and the leading coefficient is positive; so, the graph of P(x) will approach infinity as x approaches both infinity and negative infinity.”

This is very similar to how you would evaluate end behavior using limits.

Say we took:

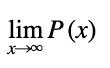
Unfortunately, we can’t literally plug infinity into P(x), so we have to think about it. What we want to know is what happens to P(x) when x gets really large in the positive direction. If you try plugging in increasingly large x-values into P(x), you will notice that it begins to grow faster and faster. This makes sense, because the term with the largest power should eventually take control of the behavior of P(x), and the other terms will have a negligible effect. Since x⁴ has the largest degree, we are essentially looking at:

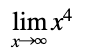
This clearly blows up to a really big number, which is consistent with our earlier finding that P(x) will approach infinity as x approaches infinity. We should also take a look at our leading coefficient, but since it is positive here, it won’t really affect our limit. However, if we had a negative leading coefficient, our values would approach negative infinity instead.

Works cited

https://web.ma.utexas.edu/users/m408n/m408c/CurrentWeb/LM2-2-9.php

https://www.cliffsnotes.com/study-guides/calculus/calculus/limits/infinite-limits

https://www.mathsisfun.com/calculus/limits-infinity.html

https://tutorial.math.lamar.edu/Classes/CalcI/LimitsAtInfinityI.aspx

https://www.varsitytutors.com/hotmath/hotmath_help/topics/end-behavior-of-a-function

Click and check out these popular articles for more information: 🙂

Calculus: Two Important Theorems – The Squeeze Theorem and Intermediate Value Theorem

Calculus: Limits and Continuity

Calculus: Limits with Explanations, Practice Questions, and Answers [AP Calculus, Calculus 101, Math]

Ectoderm vs Endoderm vs Mesoderm

Psychology 101 and the Brain: Stress – Definition, Symptoms, and Health Effects of the Fight-or-Flight Response

Circulatory System: Blood Flow Pathway Through the Heart

Circulatory System: Heart Structures and Functions

Ductus Arteriosus Vs Ductus Venosus Vs Foramen Ovale: Fetal Heart Circulation

Cardiac Arrhythmias: Definition, Types, Symptoms, and Prevention

Upper Vs Lower Respiratory System: Upper vs Lower Respiratory Tract Infections

Seven General Functions of the Respiratory System

Digestive System Anatomy: Diagram, Organs, Structures, and Functions

Kidney Embryology & Development: Easy Lesson

Psychology 101: Crowd Psychology and The Theory of Gustave Le Bon

Introduction to Evolution: Charles Darwin and Alfred Russel Wallace

Symbolism of Shoes in Dreams

All rights reserved. This essay first published on moosmosis.org or any portion thereof may not be reproduced or used in any manner whatsoever
without the express written permission of the publisher at moosmosis.org.